Class 12 RD Sharma Solutions – Chapter 7 Adjoint and Inverse of a Matrix – Exercise 7.1 | Set 2

Question 10. For the following parts of matrices verify that (AB)^-1 = B^-1A^-1.

(i) A = 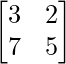and B = 

Solution:

To prove (AB)^-1= B^-1A^-1

We take LHS

AB = 

= 

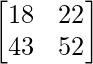
|AB| = 18 × 52 – 22 × 43

= 936 – 946 = -10

adj(AB) = 

AB^-1= adj(AB)/|AB| =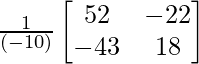 

= 

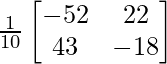
Now,

A = 

|A| = 15 – 14 = 1

adj A =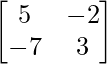 

Therefore, A^-1 = adj A/|A| =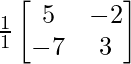 

B = 

|B| = 8 – 18 = -10

adj B =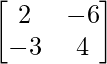 

Therefore, B^-1= adj B/|B| =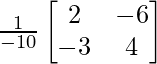 

Now, we take RHS

B^-1A^-1 =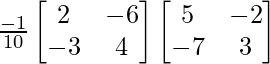 

= 

= 

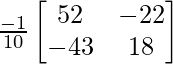
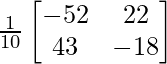
LHS = RHS 

Hence, Proved 

(ii) A = 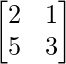and B =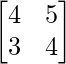 

Solution:

To prove (AB)^-1 = B^-1A^-1

We take LHS

AB =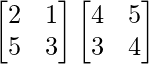 

= 

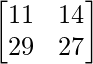
|AB| = 11 × 27 – 29 × 14

= 407 – 406 = 1

adj(AB) =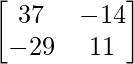 

AB^-1= adj(AB)/|AB| =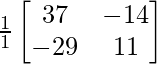 

=

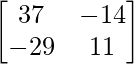
Now,

A =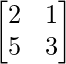 

|A| = 6 – 5 = 1

adj A=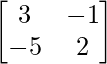

Therefore, A^-1 = adj A/|A| =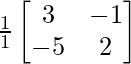 

B =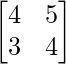 

|B| = 16 – 15 = 1

adj B =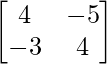

Therefore, B^-1= adj B/|B| =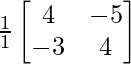 

Now, we take RHS

B^-1A^-1 =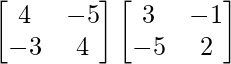 

= 

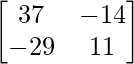
LHS = RHS 

Hence, Proved 

Question 11.  Let A = 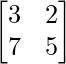and B = 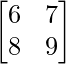. Find (AB)^-1.

Solution:

AB =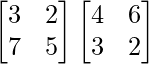

=

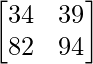
|AB| = 34 × 94 – 39 × 82 = -2

adj(AB) =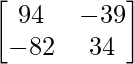 

AB^-1 = adj(AB)/|AB| =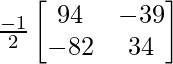 

= 

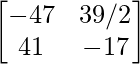
Question 12. Given A = , Compute A^-1 and show that 2A^-1 = 9I – A. 

Solution:

A =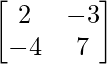

|A| = 14 – 12 = 2

adj A = 

Therefore, A^-1 = adj A/|A| =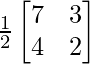 

 To show 2A^-1 = 9I – A.                   

LHS = 2 × (1/2) 

= 

Now we take RHS 

= 9I – A

=  – 

=

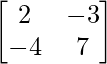
LHS = RHS

Hence Proved

Question 13. If A = , then show that A – 3I = 2(I + 3A^-1).

Solution:

Here, A = 

|A| = 4 – 10 = -6

adj A =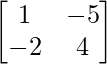

Therefore, A^-1 = adj A/|A| =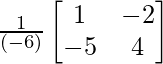 

To show, A – 3I = 2(I + 3A^-1)

Now we take LHS 

= A – 3I

= – 3

=

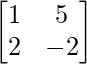
Now we take RHS 

= 2I + 6A^-1

= 2 + 6 × (1/6)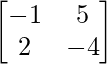

= 

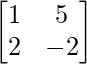
LHS = RHS 

Hence Proved        

Question 14. Find the inverse of the matrix A = 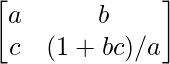and show that aA^-1 = (a² + bc + 1)I – aA.

Solution:

Here, A =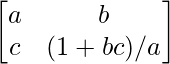 

|A| = (a + abc)/a – bc = 1

Therefore, inverse of A exists 

Cofactor of A are,

C₁₁ = (1 + bc)/a     C₁₂ = -c

C₂₁ = -b                C₂₂ = a

adj A = 

= 

= 

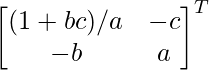
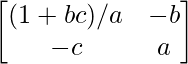
A^-1 = 1/|A|. adj A

= 1/1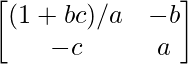 

= 

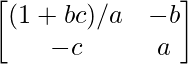
To show that 

aA^-1 = (a² + bc + 1)I – aA.

LHS = aA^-1

= a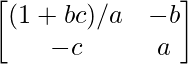

= 

RHS = (a² + bc + 1)I – a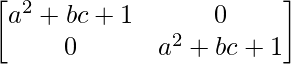A

=  – a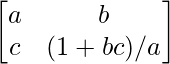

=  – 

= 

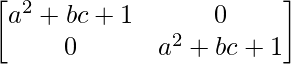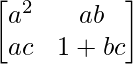
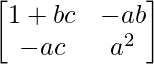
LHS = RHS 

Hence Proved         

Question 15. Given A = 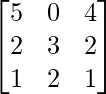, B^-1 = 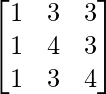, Compute (AB)^-1.

Solution:

We know (AB)^-1 = B^-1A^-1

Here, A =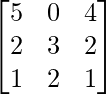 

|A| = 5(3 – 4) + 4(4 – 3) = -5 + 4 = -1 

Co-factors of A are:

C₁₁ = -1      C₁₂ = 0      C₁₃ = 1

C₂₁ = 8       C₂₂ = 1      C₂₃ = -10

C₃₁ = -12    C₃₂ = -2    C₃₃ = 15

adj A =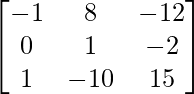 

A^-1 = 1/|A|. adj A

Hence, A^-1 =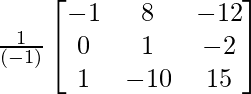 

= 

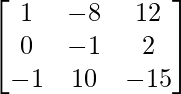
 (AB)^-1 = B^-1A^-1

= 

= 

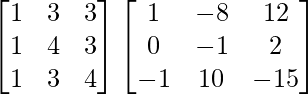
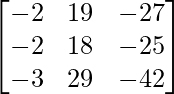
Question 16. Let F(α) = 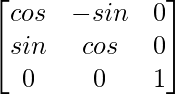 and G(β) = , Show that

(i) [F(α)]^-1 = F(-α) 

Solution:

We have F(α) =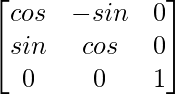 

|F(α)| = cos²α + sin²α = 1

Therefore, inverse of F(α) exists

Cofactors of F(α) are:  

C₁₁ = cosα    C₁₂ = -sinα     C₁₃ = 0

C₂₁ = sinα     C₂₂ = cosα     C₂₃ = 0

C₃₁ = 0          C₃₂ = 0           C₃₃ = 1

Adj F(α) =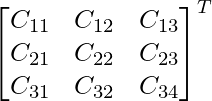 

=

=

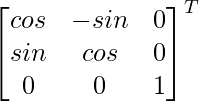
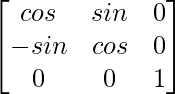
[F(α)]^-1 = 1/|F(α)|. adj F(α)

Hence, [F(α)]^-1 = 1/1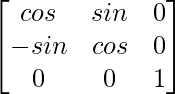

=

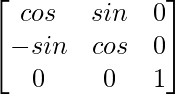
Now, F(-α) =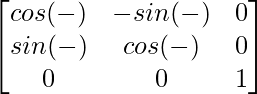 

=

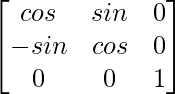
So, [F(α)]^-1 = F(-α)

Hence, Proved

(ii) [G(β)]^-1 = G(-β) 

Solution:

We have G(β) =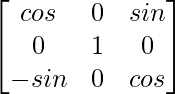 

|G(β)| = cos²β + sin²β = 1

Therefore, inverse of G(β) exists

Cofactors of G(β) are:  

C₁₁ = cosβ   C₁₂ = 0      C₁₃ = sinβ

C₂₁ = 0         C₂₂ = 1      C₂₃ = 0

C₃₁ = -sinβ   C₃₂ = 0     C₃₃ = sinβ

Adj G(β) =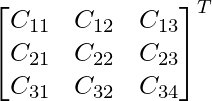 

=

=

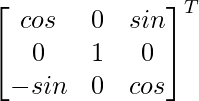
[G(β)]^-1 = 1/|G(β)|. adj G(β)

Hence, [G(β)]^-1 = 1/1

= 

Now, G(-β) =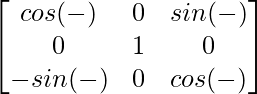

= 

So, [G(β)]^-1 = G(-β)

Hence, Proved

(iii) [F(α)G(β)]^-1 = F(-α)G(-β)

Solution:

We already know that S[G(β)]^-1 = G(-β) 

 [F(α)]^-1 = F(-α) 

Taking LHS = [F(α)G(β)]^-1

= [F(α)]^-1[G(β)]^-1

= F(-α)G(-β) = RHS 

Hence, Proved

Question 17. If A = , Verify that A² – 4A + I = O, where I =  and O = , Hence, find A^-1.

Solution: 

Here, A = 

A² =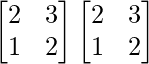 

= 

4A = 4

= 

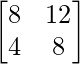
A² – 4A + I = O

=  – + 

= 

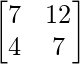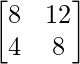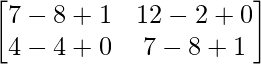
Hence, = 

Now, A² – 4A + I = O

 A² – 4A = -I

Multiplying both side by A^-1 both sides we get 

A.A(A^-1) – 4AA^-1 = -IA^-1

AI – 4I = -A^-1

A^-1 = 4I – AI    

=  – 

= 

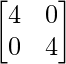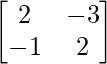
Question 18.  Show that A = 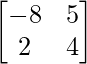 satisfies the equation A² + 4A – 42I = O. Hence, Find A^-1. 

Solution:

Here, A =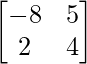 

A² =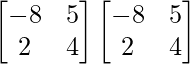 

=

=

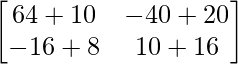
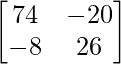
4A = 4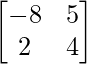

= 

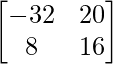
A² + 4A – 42I =  +  –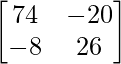 

=

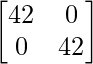
Hence, 

Now, A² + 4A – 42I = 0

⇒ A^-1A.A + 4A^-1.A – 42A^-1I = 0

⇒ IA + 4I – 42A^-1 = 0

⇒ A^-1 = 1/42 [A + 4I]

⇒ A^-1 =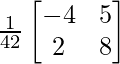 

Question 19. If A = , show that A² – 5A + 7I = O. Hence find A^-1.

Solution:

Here, A =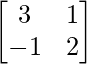 

A² =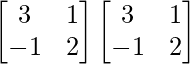 

=

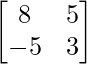
Now, A² – 5A + 7I = 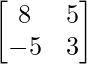 + 5 + 7

=

=

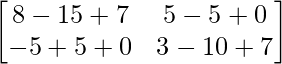
Now, A² – 5A + 7I = O

Multiplying by A^-1 both sides

⇒ A^-1AA + 5AA – 1 + 7IA^-1 = 0

⇒ A^-1 = 1/7[5I – A]

⇒ A^-1 =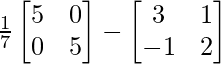 

⇒ A^-1 = 

Question 20. If A = 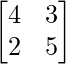, find x and y such that A² – xA + yI = O. Hence, evaluate A^-1.

Solution:

Here, A =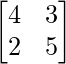 

A² =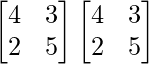 

= 

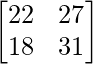
Now, A² – xA + yI = O

⇒  –   + 

= 

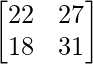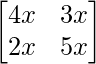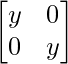
⇒ 22 – 4x + y = 0

⇒ 4x – y = 22  ………(i)

or 

18 – 2x = 0

⇒ x = 9

Putting x = 9 in eq (i)

⇒ y = 14

A² – 9A + 14I = 0

⇒ 9A = A² + 14I

⇒ 9A^-1A = A^-1AA + 14A^-1

⇒ 9I = IA + 14A^-1

⇒ A^-1 = 1/14[9I – A] = 1/14()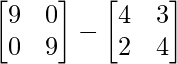

⇒ A^-1=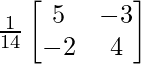 

Question 21. If A = 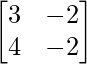, find the value of λ so that A² = λA – 2I. Hence, find A^-1. 

Solution:

Here, A =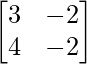 

A² =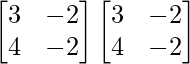 

= 

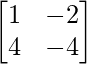
If A² = λA – 2I 

λA = A² + 2I

⇒ λ  =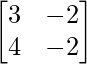 

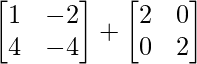
⇒ λ  =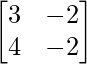 

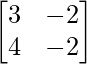
⇒ λ = 1

Now, A² = λA – 2I

Multiplying both side A^-1

⇒ A^-1AA = A^-1A – 2A^-1I

⇒ A = I – 2A^-1

⇒ 2A^-1 = I – A =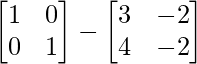 

A^-1 =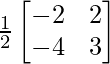 

Question 22. Show that A = 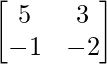 satisfies the equation x² – 3x – 7 = 0. Thus, find A^-1.

Solution:

Here, A =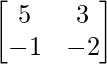 

A² =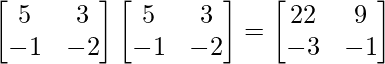 

Now, A² – 3A – 7=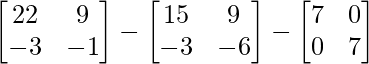 

=

We have, A² – 3A – 7 = 0

⇒ A^-1AA – 3A^-1A – 7A^-1 = 0

⇒ A-3I – 7A^-1 = 0

⇒ 7A^-1 = A – 3I

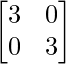
⇒ 7A^-1 =  –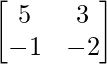 

A^-1 =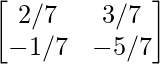 

Question 23. Show that A =  satisfies the equation x² – 12x + 1 = 0. Thus, find A^-1.

Solution:

Here, A = 

A² =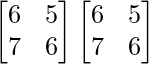 

= 

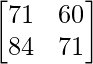
Now, A² – 12A + I = –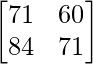 

=

We have, A² – 12A + I = 0

⇒ A – 12I + A^-1 = 0 

⇒ A^-1 = 12I – A

⇒ A^-1 =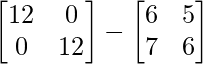 

⇒ A^-1 =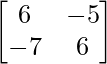 

Question 24. For the matrix A = show that A³ – 6A² + 5A + 11I₃ = O.  Hence, find A^-1.

Solution:

Here, A =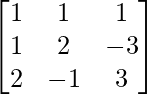

A² =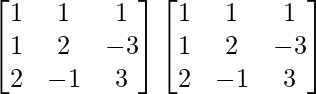 

= 

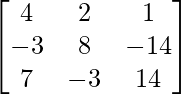
A³ =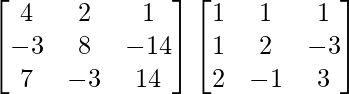 

= 

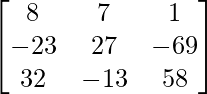
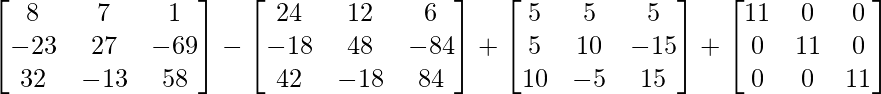
A³ – 6A² + 5A + 11I

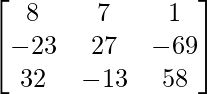=  – 6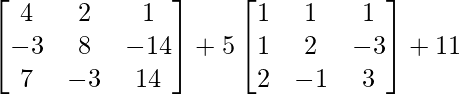 

=

= 

= 

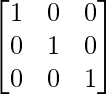
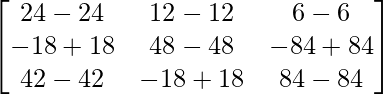
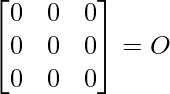
We have, A³ – 6A² + 5A + 11I = O.

⇒ A^-1(AAA) – 6A^-1(AA) + 5A^-1A + 11IA^-1 = 0

⇒ A² – 6A + 5I = -11A^-1

⇒ -11A^-1 = (A² – 6A + 5I)

=

=

=

=

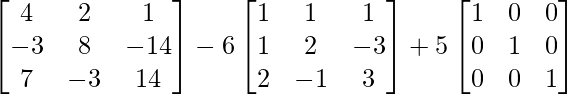
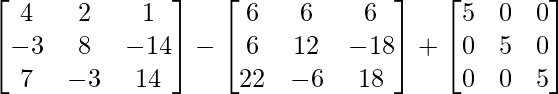
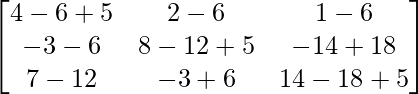
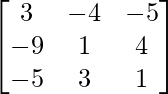
Therefore, A^-1 =