Class 12 RD Sharma Solutions – Chapter 29 The Plane – Exercise 29.9
Last Updated :
12 Oct, 2021
Question 1. Find the distance of the point 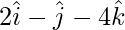 from the plane
from the plane 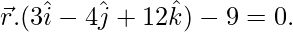
Solution:
As we know the distance of a point  from a plane
from a plane 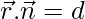 is given by:
is given by:
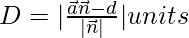
Here, 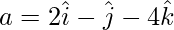 and
and 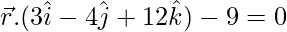 is the plane.
is the plane.
Hence, 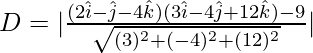
⇒ 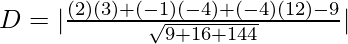
= |-47/13| units
= 47/13 units
Hence, the distance of the point from the plane is 47/13 units.
Question 2. Show that the points 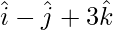 and
and  are equidistant from the plane
are equidistant from the plane 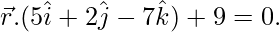
Solution:
As we know the distance of a point 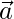 from a plane
from a plane 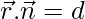 is given by:
is given by:
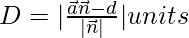
Let D1 be the distance of 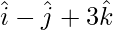 from the plane
from the plane 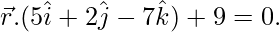
⇒ 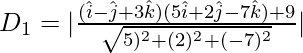
= 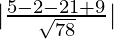
= 9/√78 units …….(1)
Now, let D2 be the distance between point  and the plane
and the plane 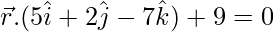 .
.
⇒ 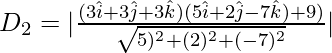
= 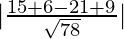
= 9/√78 units ……(2)
From eq(1) and (2), we have
The given points are equidistant from the given plane.
Question 3. Find the distance of the point (2, 3, −5) from the plane x + 2y − 2z − 9 = 0.
Solution:
As we know the distance is given by:
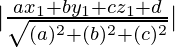
⇒ 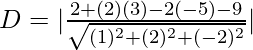
= 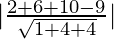
= 9/√9
D = 3 units.
Question 4. Find the equations of the planes parallel to the plane x + 2y − 2z + 8 = 0 which are at a distance of 2 units from the point (2, 1, 1).
Solution:
The equation of a plane parallel to the given plane is x + 2y − 2z + p = 0.
As we know that the distance between a point and plane is given by:
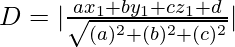
Given, D = 2 units. Hence,
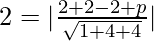
⇒ 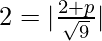
On squaring both sides, we have
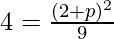
⇒ 36 = (2 + p)2
⇒ 2 + p = 6 or 2 + p = −6
⇒ p = 4 or p = −8
Hence, the equations of the required planes are:
x + 2y − 2z + 4 = 0 and x + 2y − 2z − 8 = 0.
Question 5. Show that the points (1, 1, 1) and(−3, 0, 1) are equidistant from the plane 3x + 4y − 12z +13 = 0.
Solution:
We know that the distance between a point and plane is given by:
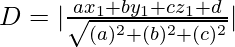
Let D1 be the distance of the point (1,1,1) from the plane.
⇒ 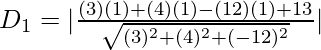
= 8/13 units
Let D2 be the distance of the point.
⇒ 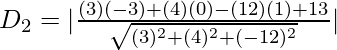
= 8/13 units
Hence, the points are equidistant from the plane.
Question 6. Find the equation of the planes parallel to the plane x − 2y + 2z − 3 = 0 which are at a unit distance from the point (2, 1, 1).
Solution:
Equation of a plane parallel to the given plane is x − 2y + 2z + p = 0.
As we know that the distance between a point and plane is given by:
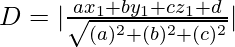
Given, D = 1 units. Hence,
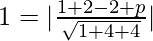
⇒ 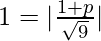
On squaring both sides, we have
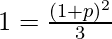
⇒ 9 = (1 + p)2
⇒ 1 + p = 3 or 1 + p = −3
⇒ p = 2 or p = −4
Hence, the equations of the required planes are:
x − 2y + 2z + 2 = 0 and x − 2y + 2z − 4 = 0.
Question 7. Find the distance of the point (2, 3, 5) from the xy-plane.
Solution:
As we know the distance of the point from the plane is given by:
D = 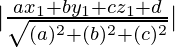
= 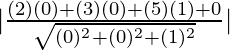
= 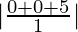
D = 5 units
Question 8. Find the distance of the point (3, 3, 3) from the plane 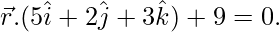
Solution:
As we know the distance of a point  from a plane
from a plane  is given by:
is given by:
D = 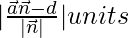
= 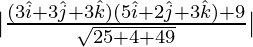
= 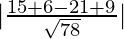
D = 9/√78 units.
Question 9. If the product of distances of the point (1, 1, 1) from the origin and the plane x − y + z + p = 0 be 5, find p.
Solution:
The distance of the point (1, 1, 1) from the origin is 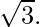
Distance of (1, 1, 1) from the plane is 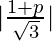
Given: 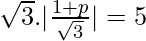
⇒ |1 + p| = 5
⇒ p = 4 or −6.
Question 10. Find an equation of the set of all points that are equidistant from the planes 3x − 4y + 12 = 6 and 4x + 3z = 7.
Solution:
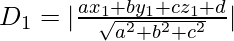

= 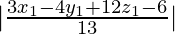
Now, 
= 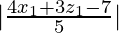
Given, D1 = D2
⇒ 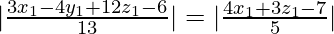
Hence, the equations become
37x1 + 20y1 − 21z1 − 61 = 0 and 67x1 + 20y1 + 99z1 − 121 = 0.
Question 11. Find the distance between the point (7, 2, 4) and the plane determined by the points A(−2, −3, 5) and C(5, 3, −3).
Solution:
The equations of the plane are given as:
−4a − 8b + 8c = 0 and 3a − 2b + 0c = 0
Solving the above set using cross multiplication method, we get
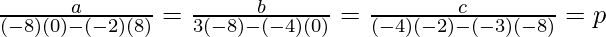
⇒ 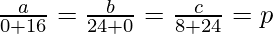
⇒ 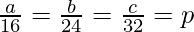
⇒ 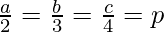
⇒ a = 2p, b = 3p, c = 4p
Thus, the equation of the plane becomes 2x + 3y + 4z − 7 = 0.
and, distance = √29 units.
Question 12. A plane makes intercepts −6, 3, 4 respectively on the coordinate axis. Find the length of the perpendicular from the origin on it.
Solution:
Since the plane makes intercepts −6, 3, 4, the equation becomes:
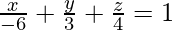
Let p be the distance of perpendicular from the origin to the plane.
⇒ 
⇒ 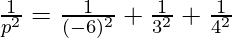
⇒ 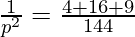
⇒ 1/p2 = 29/144
⇒ p2 = 144/29
⇒ p = 12/√29 units.
Share your thoughts in the comments
Please Login to comment...