Class 11 RD Sharma Solutions – Chapter 32 Statistics – Exercise 32.2
Last Updated :
11 Feb, 2021
Question 1. Calculate the mean deviation from the median of the following frequency distribution:
| Heights in inches | 58 | 59 | 60 | 61 | 62 | 63 | 64 | 65 | 66 |
| No. Of Students | 15 | 20 | 32 | 35 | 35 | 22 | 20 | 10 | 8 |
Solution:
Median is the middle term of the observation in ascending order,
So, Median = 61
Let us assume,
xi =Heights in inches
fi = Number of students
| xi | fi | Cumulative Frequency |
|di| = |xi – M|
= |xi – 61|
| fi |di| |
| 58 | 15 | 15 | 3 | 45 |
| 59 | 20 | 35 | 2 | 40 |
| 60 | 32 | 67 | 1 | 32 |
| 61 | 35 | 102 | 0 | 0 |
| 62 | 35 | 137 | 1 | 35 |
| 63 | 22 | 159 | 2 | 44 |
| 64 | 20 | 179 | 3 | 60 |
| 65 | 10 | 189 | 4 | 40 |
| 66 | 8 | 197 | 5 | 40 |
| | N = 197 | | | Total = 336 |
N=197
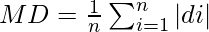
= 1/197 × 336
= 1.70
Therefore, the mean deviation is 1.70.
Question 2. The number of telephone calls received at an exchange in 245 successive on2-minute intervals is shown in the following frequency distribution:
| Number of calls | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| Frequency | 14 | 21 | 25 | 43 | 51 | 40 | 39 | 12 |
Compute the mean deviation about the median.
Solution:
Median is the middle term of the observation in ascending order,
We know, Median is the even term, (3+5)/2 = 4
So, Median = 8
Let us assume
xi =Number of calls
fi = Frequency
N = 245
| xi | fi | Cumulative Frequency |
|di| = |xi – M|
= |xi – 61|
| fi |di| |
| 0 | 14 | 14 | 4 | 56 |
| 1 | 21 | 35 | 3 | 63 |
| 2 | 25 | 60 | 2 | 50 |
| 3 | 43 | 103 | 1 | 43 |
| 4 | 51 | 154 | 0 | 0 |
| 5 | 40 | 194 | 1 | 40 |
| 6 | 39 | 233 | 2 | 78 |
| 7 | 12 | 245 | 3 | 36 |
| | Total = 245 | | | Total = 366 |
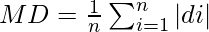
= 1/245 × 336
= 1.49
Therefore, mean deviation is 1.49.
Question 3. Calculate the mean deviation about the median of the following frequency distribution:
Solution:
Calculating the median,
We know, Number of observations, N = 50
Median = (50)/2 = 25
Therefore, the median corresponding to 25 is 13.
| xi | fi | Cumulative Frequency |
|di| = |xi – M|
= |xi – 61|
| fi |di| |
| 5 | 2 | 2 | 8 | 16 |
| 7 | 4 | 6 | 6 | 24 |
| 9 | 6 | 12 | 4 | 24 |
| 11 | 8 | 20 | 2 | 16 |
| 13 | 10 | 30 | 0 | 0 |
| 15 | 12 | 42 | 2 | 24 |
| 17 | 8 | 50 | 4 | 32 |
| | Total = 50 | | | Total = 136 |
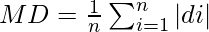
= 1/50 × 136
= 2.72
Therefore, the mean deviation is 2.72.
Question 4. Find the mean deviation from the mean for the following data:
(i)
(ii)
(iii)
Solution:
(i) We know,
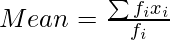
| xi | fi | Cumulative Frequency (xifi) | |di| = |xi – Mean| | fi |di| |
| 5 | 8 | 40 | 4 | 32 |
| 7 | 6 | 42 | 2 | 12 |
| 9 | 2 | 18 | 0 | 0 |
| 10 | 2 | 20 | 1 | 2 |
| 12 | 2 | 24 | 3 | 6 |
| 15 | 6 | 90 | 6 | 36 |
| | Total = 26 | Total = 234 | | Total = 88 |
Now, Mean = 234/26
= 9
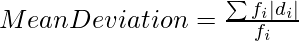
= 88/26
= 3.3
∴ The mean deviation is 3.3
(ii) We know,
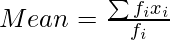
| xi | fi | Cumulative Frequency (xifi) | |di| = |xi – Mean| | fi |di| |
| 5 | 7 | 35 | 9 | 63 |
| 10 | 4 | 40 | 4 | 16 |
| 15 | 6 | 90 | 1 | 6 |
| 20 | 3 | 60 | 6 | 18 |
| 25 | 5 | 125 | 11 | 55 |
| | Total = 25 | Total = 350 | | Total = 158 |
Mean = 350/25
= 14
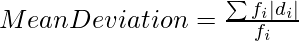
= 158/25
= 6.32
∴ The mean deviation is 6.32
(iii) We know,
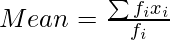
= 4000/80
= 50
| xi | fi | Cumulative Frequency (xifi) | |di| = |xi – Mean| | fi |di| |
| 10 | 4 | 40 | 40 | 160 |
| 30 | 24 | 720 | 20 | 480 |
| 50 | 28 | 1400 | 0 | 0 |
| 70 | 16 | 1120 | 20 | 320 |
| 90 | 8 | 720 | 40 | 320 |
| | Total = 80 | Total = 4000 | | Total = 1280 |
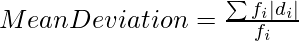
= 1280/80
= 16
∴ The mean deviation is 16
Question 5. Find the mean deviation from the median for the following data :
(i)
(ii)
| xi | 74 | 89 | 42 | 54 | 91 | 94 | 35 |
| fi | 20 | 12 | 2 | 4 | 5 | 3 | 4 |
(iii)
| Marks obtained | 10 | 11 | 12 | 14 | 15 |
| No. of students | 2 | 3 | 8 | 3 | 4 |
Solution:
(i) We know,
Number of observations, N = 21
Median = (21)/2 = 10.5
Therefore, the median corresponding to 10.5 is 27
| xi | fi | Cumulative Frequency | |di| = |xi – M| | fi |di| |
| 15 | 3 | 3 | 15 | 45 |
| 21 | 5 | 8 | 9 | 45 |
| 27 | 6 | 14 | 3 | 18 |
| 30 | 7 | 21 | 0 | 0 |
| | Total = 21 | Total = 46 | | Total = 108 |
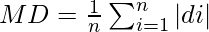
= 1/21 × 108
= 5.14
∴ The mean deviation is 5.14
(ii) We know,
Number of observations, N =50
Median = (50)/2 = 25
Therefore, the median corresponding to 25 is 74.
| xi | fi | Cumulative Frequency | |di| = |xi – M| | fi |di| |
| 74 | 20 | 4 | 39 | 156 |
| 89 | 12 | 6 | 32 | 64 |
| 42 | 2 | 10 | 20 | 80 |
| 54 | 4 | 30 | 0 | 0 |
| 91 | 5 | 42 | 15 | 180 |
| 94 | 3 | 47 | 17 | 85 |
| 35 | 4 | 50 | 20 | 60 |
| | Total = 50 | Total = 189 | | Total = 625 |
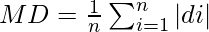
= 1/50 × 625
= 12.5
Therefore, the mean deviation is 12.5
(iii) We know,
Number of observations, N =20
Median = (20)/2 = 10
So, the median corresponding to 10 is 12.
| xi | fi | Cumulative Frequency | |di| = |xi – M| | fi |di| |
| 10 | 2 | 2 | 2 | 4 |
| 11 | 3 | 5 | 1 | 3 |
| 12 | 8 | 13 | 0 | 0 |
| 14 | 3 | 16 | 2 | 6 |
| 15 | 4 | 20 | 3 | 12 |
| | Total = 20 | | | Total = 25 |
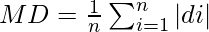
= 1/20 × 25
= 1.25
∴ The mean deviation is 1.25
Like Article
Suggest improvement
Share your thoughts in the comments
Please Login to comment...