Class 11 RD Sharma Solution – Chapter 18 Binomial Theorem- Exercise 18.2 | Set 1

Question 1. Find the 11th term from the beginning and the 11th term from the end in the expansion of (2x – 1/x²)²⁵.

Solution:

We are given, (2x – 1/x²)²⁵.
The given expression contains 25 + 1 = 26 terms.
So, the 11th term from the end is the (26 − 11 + 1) th term = 16th term from the beginning.
Hence, T₁₆ = T₁₅₊₁ = ²⁵C₁₅ (2x)^25-15 (−1/x²)¹⁵
= ²⁵C₁₅ (2¹⁰) (x)¹⁰ (−1/x³⁰)
= – ²⁵C₁₅ (2¹⁰/ x²⁰)
Now, the 11th term from the beginning is,
T₁₁ = T₁₀₊₁ = ²⁵C₁₀ (2x)^25-10 (−1/x²)¹⁰
= ²⁵C₁₀ (2¹⁵) (x)¹⁵ (1/x²⁰)
= ²⁵C₁₀ (2¹⁵/ x⁵)

Question 2. Find the 7th term in the expansion of (3x² – 1/x³)¹⁰.

Solution:

We are given, (3x² – 1/x³)¹⁰.
The 7th term of the expression is given by,
T₇ = T₆₊₁
= ¹⁰C₆ (3x²)¹⁰⁻⁶ (−1/x³)⁶
= ¹⁰C₆ (3)⁴ (x)⁸ (1/x¹⁸)
= $\frac{10×9×8×7×81}{4×3×2×x^{10}}$
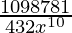
= 17010/ x¹⁰

Question 3. Find the 5th term from the end in the expansion of (3x – 1/x²)¹⁰.

Solution:

We are given, (3x – 1/x²)¹⁰
The 5th term from the end is the (11 – 5 + 1)th = 7th term from the beginning.
So, T₇ = T₆₊₁
= ¹⁰C₆ (3x)^10-6 (–1/x²)⁶
= ¹⁰C₆ (3)⁴ (x)⁴ (1/x¹²)
= $\frac{10×9×8×7×81}{4×3×2×x^8}$
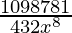
= 17010/ x⁸

Question 4. Find the 8th term in the expansion of (x^3/2 y^1/2 – x^1/2 y^3/2)¹⁰.

Solution:

We are given, (x^3/2 y^1/2 – x^1/2 y^3/2)¹⁰.
The 8th term of the expression is given by,
T₈ = T₇₊₁
= ¹⁰C₇ (x^3/2 y^1/2)^10–7 (–x^1/2y^3/2)⁷
= $\frac{-(10×9×8)}{3×2} x^{9/2} y^{3/2} (x^{7/2} y^{21/2})$
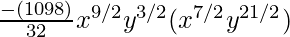
= –120 x⁸y¹²

Question 5. Find the 7th term in the expansion of (4x/5 + 5/2x)⁸.

Solution:

We are given, (4x/5 + 5/2x)⁸.
The 8th term of the expression is given by,
T₇ = T₆₊₁
= $^8C_6(\frac{4x}{5})^{8-6}(\frac{5}{2x})^6$
= $\frac{8×7×6!}{6!2!}(\frac{16x^2}{25})(\frac{125×125}{64x^6})$
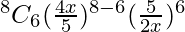
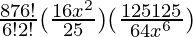
= 4375/ x⁴

Question 6. Find the 4th term from the beginning and 4th term from the end in the expansion of (x + 2/x)⁹.

Solution:

We are given, (x + 2/x)⁹.
The given expression contains 9 + 1 = 10 terms.
So, the 4th term from the end is the (10 − 4 + 1) th term = 7th term from the beginning.
Hence, T₇ = T₆₊₁ = ⁹C₆ (x)^9-6 (2/x)⁶
= $\frac{9×8×7}{3×2}(x^3)(\frac{64}{x^6})$
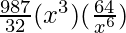
= 5376/ x³
Now, the 4th term from the beginning is,
T₄ = T₃₊₁ = ⁹C₃ (2x)^9-3 (2/x)³
= $\frac{9×8×7}{3×2}(x^6)(\frac{8}{x^3})$
= 672 x³

Question 7. Find the 4th term from the end in the expansion of (4x/5 – 5/2x)⁹.

Solution:

We are given, (4x/5 – 5/2x)⁹
The 4th term from the end is (10 − 4 + 1)th term = 7th term, from the beginning.
T₇ = T₆₊₁
= $^9C_6(\frac{4x}{5})^{9-6}(\frac{5}{2x})^6$
= $\frac{9×8×7}{3×2}(\frac{64x^3}{125})(\frac{125×125}{64x^6})$
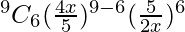
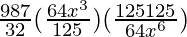
= 10500/ x³

Question 8. Find the 7th term from the end in the expansion of (2x² – 3/2x)⁸.

Solution:

We are given, (2x² – 3/2x)⁸
The 7th term from the end is (9 − 7 + 1)th term = 3rd term, from the beginning.
T₃ = T₂₊₁
= $^8C_2(2x^2)^{8-2}(\frac{-3}{2x})^2$
= $\frac{8×7}{2×1}(64x^{12})(\frac{9}{4x^2})$
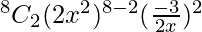
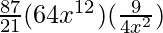
= 4032 x¹⁰

Question 9. Find the coefficient of:

(i) x¹⁰ in the expansion of (2x² – 1/x)²⁰

Solution:

We are given, (2x² – 1/x)²⁰
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_rx^n-r a^r
= ²⁰C_r (2x²)^20-r (-1/x)^r
= (-1)^r ²⁰C_r (2)^20-r x^40-2r-r
If x¹⁰ exists in the expansion, we must have,
=> 40 − 3r = 10
=> 3r = 30
=> r = 10
Coefficient of x¹⁰ = (-1)¹⁰ ²⁰C₁₀ (2)^20-10
= ²⁰C₁₀ (2)¹⁰

(ii) x⁷ in the expansion of (x – 1/x²)⁴⁰

Solution:

We are given, (x – 1/x²)⁴⁰
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= ⁴⁰C_r (x)^40-r (-1/x²)^r
= (-1)^r ⁴⁰C_r x^40-r-2r
If x⁷ exists in the expansion, we must have,
=> 40 − 3r = 7
=> 3r = 33
=> r = 11
Coefficient of x⁷ = (-1)¹¹ ⁴⁰C₁₁
= − ⁴⁰C₁₁

(iii) x^-15 in the expansion of (3x² – a/3x³)¹⁰

Solution:

We are given, (3x² – a/3x³)¹⁰
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= ¹⁰C_r (3x²)^10-r (a/3x³)^r
= (-1)^r ¹⁰C_r 3^10-r-r x^20-2r-3r a^r
If x^-15 exists in the expansion, we must have,
=> 20 − 5r = −15
=> 5r = 35
=> r = 7
Coefficient of x^-15 = (-1)⁷ ¹⁰C₇ 3^10-14 a⁷
= $-\frac{10×9×8}{3×2×9×9}a^7$
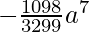
= −40a⁷/27

(iv) x⁹ in the expansion of (x² – 1/3x)⁹

Solution:

We are given, (x² – 1/3x)⁹
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= ⁹C_r (x²)^9-r (-1/3x)^r
= (-1)^r ⁹C_r x^18-2r-r (1/3)^r
If x⁹ exists in the expansion, we must have,
=> 18 − 3r = 9
=> 3r = 9
=> r = 3
Coefficient of x⁹ = (-1)³ ⁹C₃ (1/3)³
= $-\frac{9×8×7}{2×9×9}$
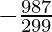
= −28/9

(v) x^m in the expansion of (x + 1/x)ⁿ

Solution:

We are given, (x + 1/x)ⁿ
We know, the (r + 1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^{n- r} (1/x^r)
= ⁿC_r x^{n- 2r}
If x^m exists in the expansion, we must have,
=> n – 2r = m
=> r = (n – m)/2
Coefficient of x^m = ⁿC_(n-m)/2
= $\frac{n!}{\left( \frac{n - m}{2} \right)! \left( \frac{n + m}{2} \right)!}$

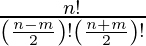
(vi) x in the expansion of (1 – 2x³ + 3x⁵) (1 + 1/x)⁸

Solution:

We are given, (1 – 2x³ + 3x⁵) (1 + 1/x)⁸
We know, the (r + 1)^th term of the expression is given by,
= (1 – 2x³ + 3x⁵) (⁸C₀ + ⁸C₁ (1/x) + ⁸C₂ (1/x²) + ⁸C₃ (1/x³) + ⁸C₄ (1/x⁴) + ⁸C₅ (1/x⁵) + ⁸C₆ (1/x⁶) + ⁸C₇ (1/x⁷) + ⁸C₈ (1/x⁸))
Here, x appear in the above expression at -2 x^{3 8}C₂ (1/x²) + 3x⁵.⁸C₄ (1/x⁴)
So, coefficient of x = 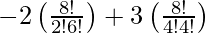$-2\left( \frac{8!}{2! 6!} \right) + 3\left( \frac{8!}{4! 4!} \right)$
= – 56 + 210
= 154

(vii) a⁵b⁷ in the expansion of (a – 2b)¹²

Solution:

We are given, (a – 2b)¹²
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= (-1)^r ¹²C_r(a)^12-r (2b)^r
If a⁵b⁷exists in the expansion, we must have,
=> 12 − r = 5
=> r = 7
Coefficient of a⁵b⁷ = (-1)^{7 12}C₇ (2)⁷
= $-\frac{12×11×10×9×8×128}{5×4×3×2}$
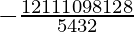
= − 101376

(viii) x in the expansion of (1 – 3x + 7 x²) (1 – x)¹⁶

Solution:

We are given, (1 – 3x + 7 x²) (1 – x)¹⁶
We know, the (r + 1)^th term of the expression is given by,
= (1 – 3x + 7x²) (¹⁶C₀ + ¹⁶C₁ (-x) + ¹⁶C₂ (-x)² + ¹⁶C₃ (-x)³+ ¹⁶C₄ (-x)⁴ + ¹⁶C₅ (-x)⁵ + ¹⁶C₆ (-x)⁶ + ¹⁶C₇ (-x)⁷ + ¹⁶C₈ (-x)⁸ + ¹⁶C₉ (-x)⁹+ ¹⁶C₁₀ (-x)¹⁰ + ¹⁶C₁₁ (-x)¹¹ + ¹⁶C₁₂ (-x)12 + ¹⁶C₁₃(-x)¹³ + ¹⁶C₁₄ (-x)¹⁴ + ¹⁶C₁₅ (-x)¹⁵ + ¹⁶C₁₆ (-x)¹⁶)
Here, x appear in the above expression at ¹⁶C₁ (-x) – 3x ¹⁶C₀
So, the coefficient of x = 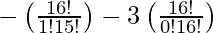$-\left( \frac{16!}{1! 15!} \right) - 3\left( \frac{16!}{0! 16!} \right)$
= – 16 – 3
= – 19

Question 10. Which term in the expansion of $\left[\left(\frac{x}{\sqrt{y}}\right)^{\frac{1}{3}}+\left(\frac{x}{\sqrt[3]{y}}\right)^{\frac{1}{2}}\right]^{21}$ contains x and y to one and the same power.

Solution:

We are given, $\left[\left(\frac{x}{\sqrt{y}}\right)^{\frac{1}{3}}+\left(\frac{x}{\sqrt[3]{y}}\right)^{\frac{1}{2}}\right]^{21}$
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= $^{21}C_r\left[(\frac{x}{\sqrt{y}})^{\frac{1}{3}}\right]^{21-r}\left[(\frac{y}{\sqrt[3]x})^{\frac{1}{2}}\right]^{r}$
= ²¹C_r x^7-r/2 y^2r/3-7/2
If x and y have same power, we must have,
=> 7 − r/2 = 2r/3 − 7/2
=> 7r/6 = 21/2
=> r = 9
Hence the required term is 9 + 1 = 10^th term.

Question 11. Does the expansion of (2x² – 1/x)²⁰ contain any term involving x⁹?

Solution:

We are given, (2x² – 1/x)²⁰
We know, the (r+1)^th term of the expression is given by,
T_r+1= ⁿC_r x^n-r a^r
= ²⁰C_r (2x²)^20-r (1/x)^r
= ²⁰C_r (2)^20-r x^40-2r-r
If x⁹ exists in the expansion, we must have,
=> 40 − 3r = 9
=> 3r = 31
=> r = 31/3
It is not possible, since r is not an integer.
Hence, there is no term with x⁹ in the given expansion.

Question 12. Show that the expansion of (x² + 1/x)¹² does not contain any term involving x^-1.

Solution:

We have, (x² + 1/x)¹²
We know, the (r+1)^th term of the expression is given by,
T_r+1 = ⁿC_r x^n-r a^r
= ¹²C_r (x²)^12-r (1/x)^r
= ¹²C_r x^24-2r-r
For this term to contain x^-1, we must have
=> 24 – 3r = −1
=> 3r = 24 + 1
=> 3r = 25
=> r = 25/3
It is not possible, since r is not an integer.
Hence, there is no term with x^-1 in the given expansion.

Question 13. Find the middle term in the expansion of:

(i) (2/3x – 3/2x)²⁰

Solution:

We have,
(2/3x – 3/2x)²⁰ where, n = 20 (which is an even number)
So, the middle term is (n/2 + 1) = (20/2 + 1) = (10 + 1) = 11^th term
Now,
T₁₁ = T₁₀₊₁
= ²⁰C₁₀ (2/3x)^20-10 (3/2x)¹⁰
= ²⁰C₁₀ (2¹⁰/3¹⁰) × (310/210) x^10-10
= ²⁰C₁₀

(ii) (x² – 2/x)¹⁰

Solution:

We have,
(x² – 2/x)¹⁰ where, n = 10 (which is an even number)
So, the middle term is (n/2 + 1) = (10/2 + 1) = (5 + 1) = 6^th term
Now,
T₆ = T₅₊₁
= ¹⁰C₅ (x²)^10-5 (-2/x)⁵
= $-\frac{10×9×8×7×6}{5×4×3×2×1}×32x^5$
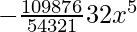
= − 8064 x⁵

(iii) (x/a – a/x)¹⁰

Solution:

We have,
(x/a – a/x)¹⁰ where, n = 10 (even number).
So the middle term is (n/2 + 1) = (10/2 + 1) = (5 + 1) = 6^th term
Now,
T₆ = T₅₊₁
= ¹⁰C₅ (x/a)^10-5 (-a/x)⁵
= $-\frac{10×9×8×7×6}{5×4×3×2×1}$
= −252

Last Updated : 14 Jul, 2021

Chapter 1: Sets

Chapter 2: Relations

Chapter 3: Functions

Chapter 4: Measurement of Angles

Chapter 5: Trigonometric Functions

Chapter 6: Graphs of Trigonometric Functions

Chapter 7: Trigonometric Ratios of Compound Angles

Chapter 8: Transformation Formulae

Chapter 9: Trigonometric Ratios of Multiple and Sub Multiple Angles

Chapter 10: Sine and Cosine Formulae and their Applications

Chapter 11: Trigonometric Equations

Chapter 12: Mathematical Induction

Chapter 13: Complex Numbers

Chapter 15: Linear Inequations

Chapter 16: Permutations

Chapter 17: Combinations

Chapter 18: Binomial Theorem

Chapter 19: Arithmetic Progressions

Chapter 20: Geometric Progressions

Chapter 21: Some Special Series

Chapter 22: Brief Review of Cartesian System of Rectangular Coordinates

Chapter 23: The Straight Lines

Chapter 24: The Circle

Chapter 25: Parabola

Chapter 26: Ellipse

Chapter 27: Hyperbola

Chapter 28: Introduction to 3D Coordinate Geometry

Chapter 29: Limits

Chapter 30: Derivatives

Chapter 31: Mathematical Reasoning

Chapter 32: Statistics

Chapter 33: Probability

Chapter 1: Sets

Chapter 2: Relations

Chapter 3: Functions

Chapter 4: Measurement of Angles

Chapter 5: Trigonometric Functions

Chapter 6: Graphs of Trigonometric Functions

Chapter 7: Trigonometric Ratios of Compound Angles

Chapter 8: Transformation Formulae

Chapter 9: Trigonometric Ratios of Multiple and Sub Multiple Angles

Chapter 10: Sine and Cosine Formulae and their Applications

Chapter 11: Trigonometric Equations

Chapter 12: Mathematical Induction

Chapter 13: Complex Numbers

Chapter 15: Linear Inequations

Chapter 16: Permutations

Chapter 17: Combinations

Chapter 18: Binomial Theorem

Chapter 19: Arithmetic Progressions

Chapter 20: Geometric Progressions

Chapter 21: Some Special Series

Chapter 22: Brief Review of Cartesian System of Rectangular Coordinates

Chapter 23: The Straight Lines

Chapter 24: The Circle

Chapter 25: Parabola

Chapter 26: Ellipse

Chapter 27: Hyperbola

Chapter 28: Introduction to 3D Coordinate Geometry

Chapter 29: Limits

Chapter 30: Derivatives

Chapter 31: Mathematical Reasoning

Chapter 32: Statistics

Chapter 33: Probability

Class 11 RD Sharma Solution – Chapter 18 Binomial Theorem- Exercise 18.2 | Set 1

Question 1. Find the 11th term from the beginning and the 11th term from the end in the expansion of (2x – 1/x2)25.

Question 2. Find the 7th term in the expansion of (3x2 – 1/x3)10.

Question 3. Find the 5th term from the end in the expansion of (3x – 1/x2)10.

Question 4. Find the 8th term in the expansion of (x3/2 y1/2 – x1/2 y3/2)10.

Question 5. Find the 7th term in the expansion of (4x/5 + 5/2x)8.

Question 6. Find the 4th term from the beginning and 4th term from the end in the expansion of (x + 2/x)9.

Question 7. Find the 4th term from the end in the expansion of (4x/5 – 5/2x)9.

Question 8. Find the 7th term from the end in the expansion of (2x2 – 3/2x)8.

Question 9. Find the coefficient of:

(i) x10 in the expansion of (2x2 – 1/x)20

(ii) x7 in the expansion of (x – 1/x2)40

(iii) x-15 in the expansion of (3x2 – a/3x3)10

(iv) x9 in the expansion of (x2 – 1/3x)9

(v) xm in the expansion of (x + 1/x)n

(vi) x in the expansion of (1 – 2x3 + 3x5) (1 + 1/x)8

Question 1. Find the 11th term from the beginning and the 11th term from the end in the expansion of (2x – 1/x²)²⁵.

Question 2. Find the 7th term in the expansion of (3x² – 1/x³)¹⁰.

Question 3. Find the 5th term from the end in the expansion of (3x – 1/x²)¹⁰.

Question 4. Find the 8th term in the expansion of (x^3/2 y^1/2 – x^1/2 y^3/2)¹⁰.

Question 5. Find the 7th term in the expansion of (4x/5 + 5/2x)⁸.

Question 6. Find the 4th term from the beginning and 4th term from the end in the expansion of (x + 2/x)⁹.

Question 7. Find the 4th term from the end in the expansion of (4x/5 – 5/2x)⁹.

Question 8. Find the 7th term from the end in the expansion of (2x² – 3/2x)⁸.

(i) x¹⁰ in the expansion of (2x² – 1/x)²⁰

(ii) x⁷ in the expansion of (x – 1/x²)⁴⁰

(iii) x^-15 in the expansion of (3x² – a/3x³)¹⁰

(iv) x⁹ in the expansion of (x² – 1/3x)⁹

(v) x^m in the expansion of (x + 1/x)ⁿ

(vi) x in the expansion of (1 – 2x³ + 3x⁵) (1 + 1/x)⁸

(vii) a⁵b⁷ in the expansion of (a – 2b)¹²

(viii) x in the expansion of (1 – 3x + 7 x²) (1 – x)¹⁶

Question 10. Which term in the expansion of $\left[\left(\frac{x}{\sqrt{y}}\right)^{\frac{1}{3}}+\left(\frac{x}{\sqrt[3]{y}}\right)^{\frac{1}{2}}\right]^{21}$ contains x and y to one and the same power.

Question 11. Does the expansion of (2x² – 1/x)²⁰ contain any term involving x⁹?

Question 12. Show that the expansion of (x² + 1/x)¹² does not contain any term involving x^-1.

(i) (2/3x – 3/2x)²⁰

(ii) (x² – 2/x)¹⁰

(iii) (x/a – a/x)¹⁰