Class 10 RD Sharma Solutions – Chapter 4 Triangles – Exercise 4.6 | Set 2

Question 11. The areas of two similar triangles are 121 cm² and 64 cm² respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.

Solution:

Let us consider ∆ABC and ∆DEF, AL and DM are the medians of ∆ABC and ∆DEF 

It is given that the area of ∆ABC = 121 cm² and area of ∆DEF = 64 cm²

AL = 12.1 cm

Let us assume DM = x cm

Given that, ∆ABC ~ ∆DEF

So, 

ar(∆ABC)/ar(∆DEF) = AL²/DM²

= 121/64 = (12.1)²/x²

11/8 = 12.1/x 

⇒ x = (8 × 12.1)/11 = 8.8

Hence, the median of the second triangle is 8.8cm 

Question 12. In ∆ABC ~ ∆DEF such that AB = 5 cm and (∆ABC) = 20 cm² and area (∆DEF) = 45 cm², determine DE.

Solution:

Given that,  

area (∆ABC) = 20 cm²

area (∆DEF) = 45 cm²

AB = 5 cm

Let us consider DE = x cm

Also, given that ∆ABC ~ ∆DEF

ar(∆ABC)/ar(∆DEF) = AB²/DE²

⇒20/45 = (5)²/x²

⇒20/45 = 25/x² 

⇒x² = (25 × 45)/20 = 225/4 = (15/2)²

x = 15/2 = 7.5 

DE = 7.5cm

Question 13. In ∆ABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divide ∆ABC into two parts equal in area. Find BP/AB.

Solution:

It is given that, in ∆ABC, PQ || BC and line PQ divide the ∆ABC into two parts 

∆APQ and trap. BPQC equally

i.e., area ∆APQ = area BPQC

Now we have to find BP/AB.

As we know that PQ||BC

So, ∆APQ ∼ ∆ABC

⇒ ar.(∆APQ)/ar.(∆ABC) = AP²/AB²

⇒ ar.(∆ABC)/ar.(∆APQ) = AB²/AP²

2/1 = AB²/AP²

{area ∆APQ = area trap. BPQC

 Area ∆ABC = 2area (∆APQ)}

⇒ AB/AP = √2/1 

⇒√2 AP = AB = AP + PB

⇒√2AP – AP = PB

⇒(√2 – 1)AP = PB

BP/AP = (√2 – 1)/1

Question 14. The areas of two similar triangles ABC and PQR are in the ratio 9 : 16. If BC = 4.5 cm, find the length of QR. 

Solution:

Given that, area (∆ABC) : area (∆PQR) = 9 : 16

∆ABC ~ ∆PQR

and BC = 4.5 cm

Let us considered QR = x cm

As we know that ∆ABC ~ ∆PQR

ar.(∆ABC)/ar.(∆PQR) = BC²/QR² ⇒ 9/16 = (4.5)²/x²

⇒ (3/4)² = (4.5/x)² ⇒ 4.5/x = 3/4

x = (4.5 × 4)/3 = 60

Hence, the length of QR is 6cm

Question 15. ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 cm, prove that area of ∆APQ is one sixteenth of the area of ∆ABC.

Solution:

Given that, in ∆ABC, P and Q are two points on line AB and AC 

AP = 1 cm, PB = 3 cm, AQ = 1.5 cm and QC = 4.5 cm

Now, AP/PB = 1/3 and AQ/QC = 1.5/4.5 = 1/3

In ∆APQ and ∆ABC

AP/PB = AQ/QC

PQ||BC

Hence, ∆APQ ∼ ∆ABC

So, ar.(∆APQ)/ar.(∆ABC) = AP²/PB² = AP²/(AP + PB)²

 ar.(∆APQ)/ar.(∆ABC) = 1²/(1 + 3)² = 1/16

Hence, area of ∆APQ = 1/16 of area of ∆ABC

Question 16. If D is a point on the side AB of ∆ABC such that AD : DB = 3 : 2 and E is a point on BC such that DE || AC. Find the ratio of areas of ∆ABC and ∆BDE. 

Solution:

Given that in ∆ABC, D is a point on AB such that AD : DB = 3 : 2

DE||AC

In ∆BDE and ∆ABC

∠BDE = ∠A

∠DBE = ∠ABC

So, by AA, ∆BED ∼ ∆ABC

Therefore, ar.(∆ABC)/ar.(∆BDE) = AB²/BD² = (BD + AD)²/BD²

= (2 + 3)²/2² = 5²/2² = 25/4

Hence, the ratio of areas of ∆ABC and ∆BDE is 25:4

Question 17. If ∆ABC and ∆BDE are equilateral triangles, where D is the mid point of BC, find the ratio of areas of ∆ABC and ∆BDE.

Solution:

Given that ∆ABC and ∆DBE are equilateral triangles, where D is mid point of BC

So, BD = 1/2BC

Now area of ∆ABC

√3/4(side)² = √3/4BC²

and area of ∆DBE

√3/4(side)² = √3/4BD²

√3/4(side)² = √3/4(1/2BC)²

√3/4(side)² = √3/16(BC)²

So, the ratio between areas is

= area(∆ABC)/area(∆DBE) =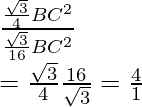

Hence, the ratio of areas of ∆ABC and ∆BDE is 4:1

Question 18. Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.

Solution:

Let us consider two triangles, ∆ABC and ∆XYZ and these triangles have equal vertical angle, i.e., ∠A and ∠X

And AD and XO is the heights of these triangles.

So, ∆ABC/∆XYZ = AB/AC = XY/XZ

In ∆ABC and ∆XYZ 

∠A = ∠X

AB/AC = XY/XZ

So, by SAS

∆ABC ~ ∆XYZ

So, ar(∆ABC)/ar(∆XYZ) = AD²/XO²

As we know that ar(∆ABC)/ar(∆XYZ) = 36/25

So, 

36/25 = AD²/XO²

6/5 = AD/XO

Hence, the ratio of their corresponding heights is 6:5

Question 19. In the figure, ∆ABC and ∆DBC are on the same base BC. If AD and BC intersect at O, prove that 

Solution:

Given that two ∆ABC and ∆DBC are on the same base BC as shown in the given figure

AC and BD intersect each other at O

Now, Draw AL ⊥ BC and DM ⊥BC

Prove: 

Proof: 

In ∆ALO and ∆DMO,

∠L =∠M = 90°

∠AOL = ∠DOM [Vertically opposite angles]

So, by AA, ∆ALO ∼ ∆DMO       

So, AL/DM = AO/DO 

Now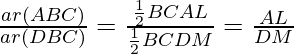 

But AL/DM = AO/DO      (Proved above)

So, 

Hence proved

Question 20. ABCD is a trapezium in which AB || CD. The diagonals AC and BD intersect at O. Prove that

(i) ∆AOB ~ ∆COD

(ii) If OA = 6 cm, OC = 8 cm, find

(a)ar(∆AOB)/ar(∆COD)        (b) ar(∆AOD)/ar(∆COD)

Solution:

Given that ABCD is a trapezium in which AB || CD and the diagonals AC and BD intersect at O

Now, in the figure from point D, draw DL⊥AC

(i) In ∆AOB and ∆COD

∠AOB =∠COD            [Vertically opposite angles]

∠OAB =∠OCD           [Alternate angles]

So, by AA criterion

∆AOB ~ ∆COD         

(ii) Given that OA = 6 cm, OC = 8 cm

As we know that ∆AOB ~ ∆COD     

So, OA/OC = OB/OD = AB/CD

(a) ar(∆AOB)/ar(∆COD) = AO²/OC²

= 6²/8² = 36/64 = 9/16 

Therefore, ar(∆AOB)/ar(∆COD) = 9/16 

(b) As we know that ∆AOD and ∆COD have their bases on the same line and their vertex A is common

Therefore,  ar(∆AOD)/ar(∆COD) = AO/OC = 6/8 = 3/4   

Question 21. In ∆ABC, P divides the side AB such that AP : PB = 1 : 2. Q is a point in AC such that PQ || BC. Find the ratio of the areas of ∆APQ and trapezium BPQC.

Solution:

Given that, ABC is a triangle, in which P divides the side AB such that 

AP : PB = 1 : 2. Q is a point in AC such that PQ || BC

In ∆APQ and ∆ABC

∠APQ = ∠B

∠PAQ = ∠BAC

So, by AA criterion

∆APQ ∼ ∆ABC

So, 

ar(∆APQ)/ar(∆ABC) = (AP)²/(AB)²

ar(∆APQ)/ar(∆ABC) = (1)²/(1 + 2)² = (1)²/(3)² = 1/9

9 ar(∆APQ) = ar(∆ABC)

9 ar(∆APQ) = ar(∆APQ) + ar(trap. BPQC)

9 ar(∆APQ) = ar(trap BPQC)

ar(∆APQ)/ar(trap BPQC) = 1/9

Hence, the ratio of the areas of ∆APQ and trapezium BPQC is 1:9

Question 22. AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (∆ADE) : Area (∆ABC) = 3 : 4. 

Solution:

Given that AD is an altitude of an equilateral triangle ABC. 

On AD as base, another equilateral triangle ADE is constructed

Prove: Area (∆ADE) : Area (∆ABC) = 3 : 4

Proof:

Area of ∆ABC = √3/4 BC²

and AD = √3/2 BC 

Area of ∆ADE = √3/4 AD²

= √3/4 (√3/2 BC)² = 3√3/16 BC²

So, the ratio of area (∆ADE):area(∆ABC) = 3√3/16 BC² : √3/4 BC²

= 3/4:1 = 3:4