Check if a graphs has a cycle of odd length

Given a graph, the task is to find if it has a cycle of odd length or not.

The idea is based on an important fact that a graph does not contain a cycle of odd length if and only if it is Bipartite, i.e., it can be colored with two colors.

It is obvious that if a graph has an odd length cycle then it cannot be Bipartite. In Bipartite graph there are two sets of vertices such that no vertex in a set is connected with any other vertex of the same set). For a cycle of odd length, two vertices must of the same set be connected which contradicts Bipartite definition.

Let us understand converse, if a graph has no odd cycle then it must be Bipartite. Below is a induction based proof of this taken from http://infohost.nmt.edu/~math/faculty/barefoot/Math321Spring98/BipartiteGraphsAndEvenCycles.html

Assume that (X, Y) is a bipartition of G and let C = u₁, u₂, . . . , u_k be a cycle of G, where u₁ is in the vertex set X (abbreviated u₁ ? X). If u_{1 ?}X then u_{2 ?} Y, . . . and, in general, u_{2j+1 ?}X and u_{2i ?}Y. Since C is a cycle, u_{k ?}Y, so that k = 2s for some positive integer s. Therefore cycle C is even.

Assume that graph G has no odd cycles. It will be shown that such a graph is bipartite. The proof is induction on the number of edges. The assertion is clearly true for a graph with at most one edge. Assume that every graph with no odd cycles and at most q edges is bipartite and let G be a graph with q + 1 edges and with no odd cycles. Let e = uv be an edge of G and consider the graph H = G – uv. By induction, H has a bipartition (X, Y). If e has one end in X and the other end in Y then (X, Y) is a bipartition of G. Hence, assume that u and v are in X. If there were a path, P, between u and v in H then the length of P would be even. Thus, P + uv would be an odd cycle of G. Therefore, u and v must be in lie in different “pieces” or components of H. Thus, we have:

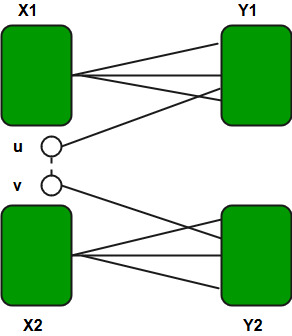
where X = X1 & X2 and Y = Y1 ? Y2. In this case it is clear that (X1 ? Y2, X2 ? Y1) is a bipartition of G.

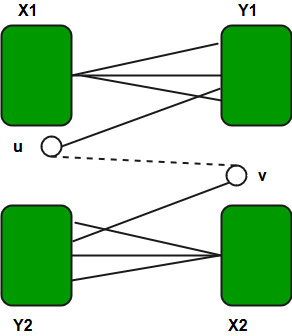
Therefore we conclude that every graph with no odd cycles is bipartite. One can construct a bipartition as follows: 

1. Choose an arbitrary vertex x₀ and set X₀ = {x₀}. 
2. Let Y₀ be the set of all vertices adjacent to x₀ and iterate steps 3-4. 
3. Let X_k be the set of vertices not chosen that are adjacent to a vertex of Y_k-1. 
4. Let Y_k be the set of vertices not chosen that are adjacent to a vertex of X_k-1. 
5. If all vertices of G have been chosen then 

X = X₀ ? X₁ ? X₂ ?. . . and Y = Y₀ ? Y₁ ? Y₂ ? . . .

Below is code to check if a graph has odd cycle or not. The code basically checks whether graph is Bipartite. 

C++

// C++ program to find out whether a given graph is
// Bipartite or not
#include <bits/stdc++.h>
#define V 4
using namespace std;

// This function returns true if graph G[V][V] contains
// odd cycle, else false
bool containsOdd(int G[][V], int src)
{
    // Create a color array to store colors assigned
    // to all vertices. Vertex number is used as index
    // in this array. The value '-1' of  colorArr[i]
    // is used to indicate that no color is assigned to
    // vertex 'i'.  The value 1 is used to indicate first
    // color is assigned and value 0 indicates second
    // color is assigned.
    int colorArr[V];
    for (int i = 0; i < V; ++i)
        colorArr[i] = -1;

    // Assign first color to source
    colorArr[src] = 1;

    // Create a queue (FIFO) of vertex numbers and
    // enqueue source vertex for BFS traversal
    queue <int> q;
    q.push(src);

    // Run while there are vertices in queue (Similar to BFS)
    while (!q.empty())
    {
        // Dequeue a vertex from queue
        int u = q.front();
        q.pop();

        // Return true if there is a self-loop
        if (G[u][u] == 1)
           return true;

        // Find all non-colored adjacent vertices
        for (int v = 0; v < V; ++v)
        {
            // An edge from u to v exists and destination
            // v is not colored
            if (G[u][v] && colorArr[v] == -1)
            {
                // Assign alternate color to this adjacent
                // v of u
                colorArr[v] = 1 - colorArr[u];
                q.push(v);
            }

            // An edge from u to v exists and destination
            // v is colored with same color as u
            else if (G[u][v] && colorArr[v] == colorArr[u])
                return true;
        }
    }

    // If we reach here, then all adjacent
    // vertices can be colored with alternate
    // color
    return false;
}

// Driver program to test above function
int main()
{
    int G[][V] = {{0, 1, 0, 1},
        {1, 0, 1, 0},
        {0, 1, 0, 1},
        {1, 0, 1, 0}
    };

    containsOdd(G, 0) ? cout << "Yes" : cout << "No";
    return 0;
}

Java

// JAVA Code For Check if a graphs has a cycle
// of odd length
import java.util.*;

class GFG {

    public static int V =4;

    // This function returns true if graph G[V][V]
    // contains odd cycle, else false
    public static boolean containsOdd(int G[][], int src)
    {
        // Create a color array to store colors assigned
        // to all vertices. Vertex number is used as
        // index in this array. The value '-1' of
        // colorArr[i] is used to indicate that no color
        // is assigned to vertex 'i'.  The value 1 is
        // used to indicate first color is assigned and
        // value 0 indicates second color is assigned.
        int colorArr[] = new int[V];
        for (int i = 0; i < V; ++i)
            colorArr[i] = -1;

        // Assign first color to source
        colorArr[src] = 1;

        // Create a queue (FIFO) of vertex numbers and
        // enqueue source vertex for BFS traversal
        LinkedList<Integer> q = new LinkedList<Integer>();
        q.add(src);

        // Run while there are vertices in queue
        // (Similar to BFS)
        while (!q.isEmpty())
        {
            // Dequeue a vertex from queue
            int u = q.peek();
            q.pop();

            // Return true if there is a self-loop
            if (G[u][u] == 1)
               return true;

            // Find all non-colored adjacent vertices
            for (int v = 0; v < V; ++v)
            {
                // An edge from u to v exists and
                // destination v is not colored
                if (G[u][v] == 1 && colorArr[v] == -1)
                {
                    // Assign alternate color to this
                    // adjacent v of u
                    colorArr[v] = 1 - colorArr[u];
                    q.push(v);
                }

                // An edge from u to v exists and
                // destination v is colored with same
                // color as u
                else if (G[u][v] == 1 && colorArr[v] ==
                                          colorArr[u])
                    return true;
            }
        }

        // If we reach here, then all adjacent
        // vertices can be colored with alternate
        // color
        return false;
    }

    /* Driver program to test above function */
    public static void main(String[] args)
    {

        int G[][] = {{0, 1, 0, 1},
                     {1, 0, 1, 0},
                     {0, 1, 0, 1},
                     {1, 0, 1, 0}};

           if (containsOdd(G, 0))
               System.out.println("Yes") ;
            else
                   System.out.println("No");
    }
}

// This code is contributed by Arnav Kr. Mandal.

Python3

# Python3 program to find out whether
# a given graph is Bipartite or not
import queue

# This function returns true if graph
# G[V][V] contains odd cycle, else false
def containsOdd(G, src):
    global V

    # Create a color array to store
    # colors assigned to all vertices.
    # Vertex number is used as index
    # in this array. The value '-1' of
    # colorArr[i] is used to indicate
    # that no color is assigned to vertex
    # 'i'. The value 1 is used to indicate
    # first color is assigned and value 0
    # indicates second color is assigned.
    colorArr = [-1] * V

    # Assign first color to source
    colorArr[src] = 1

    # Create a queue (FIFO) of vertex
    # numbers and enqueue source vertex
    # for BFS traversal
    q = queue.Queue()
    q.put(src)

    # Run while there are vertices in
    # queue (Similar to BFS)
    while (not q.empty()):

        # Dequeue a vertex from queue
        u = q.get()

        # Return true if there is a self-loop
        if (G[u][u] == 1):
            return True

        # Find all non-colored adjacent vertices
        for v in range(V):

            # An edge from u to v exists and
            # destination v is not colored
            if (G[u][v] and colorArr[v] == -1):

                # Assign alternate color to this
                # adjacent v of u
                colorArr[v] = 1 - colorArr[u]
                q.put(v)

            # An edge from u to v exists and
            # destination v is colored with
            # same color as u
            elif (G[u][v] and
                  colorArr[v] == colorArr[u]):
                return True

    # If we reach here, then all
    # adjacent vertices can be
    # colored with alternate color
    return False

# Driver Code
V = 4
G = [[0, 1, 0, 1],
     [1, 0, 1, 0],
     [0, 1, 0, 1],
     [1, 0, 1, 0]]

if containsOdd(G, 0):
    print("Yes")
else:
    print("No")

# This code is contributed by PranchalK

C#

// C# Code For Check if a graphs has a cycle
// of odd length
using System;
using System.Collections.Generic;

class GFG
{

    public static int V = 4;

    // This function returns true if graph G[V,V]
    // contains odd cycle, else false
    public static bool containsOdd(int [,]G, int src)
    {
        // Create a color array to store colors assigned
        // to all vertices. Vertex number is used as
        // index in this array. The value '-1' of
        // colorArr[i] is used to indicate that no color
        // is assigned to vertex 'i'. The value 1 is
        // used to indicate first color is assigned and
        // value 0 indicates second color is assigned.
        int []colorArr = new int[V];
        for (int i = 0; i < V; ++i)
            colorArr[i] = -1;

        // Assign first color to source
        colorArr[src] = 1;

        // Create a queue (FIFO) of vertex numbers and
        // enqueue source vertex for BFS traversal
        Queue<int> q = new Queue<int>();
        q.Enqueue(src);

        // Run while there are vertices in queue
        // (Similar to BFS)
        while (q.Count != 0)
        {
            // Dequeue a vertex from queue
            int u = q.Peek();
            q.Dequeue();

            // Return true if there is a self-loop
            if (G[u, u] == 1)
            return true;

            // Find all non-colored adjacent vertices
            for (int v = 0; v < V; ++v)
            {
                // An edge from u to v exists and
                // destination v is not colored
                if (G[u, v] == 1 && colorArr[v] == -1)
                {
                    // Assign alternate color to this
                    // adjacent v of u
                    colorArr[v] = 1 - colorArr[u];
                    q.Enqueue(v);
                }

                // An edge from u to v exists and
                // destination v is colored with same
                // color as u
                else if (G[u,v] == 1 && colorArr[v] ==
                                        colorArr[u])
                    return true;
            }
        }

        // If we reach here, then all adjacent
        // vertices can be colored with alternate
        // color
        return false;
    }

    /* Driver code */
    public static void Main()
    {

        int [,]G = {{0, 1, 0, 1},
                    {1, 0, 1, 0},
                    {0, 1, 0, 1},
                    {1, 0, 1, 0}};

        if (containsOdd(G, 0))
            Console.WriteLine("Yes") ;
            else
                Console.WriteLine("No");
    }
}

// This code has been contributed by 29AjayKumar

Javascript

<script>


// JavaScript Code For Check if a graphs has a cycle
// of odd length

var V = 4;

// This function returns true if graph G[V,V]
// contains odd cycle, else false
function containsOdd(G, src)
{
    // Create a color array to store colors assigned
    // to all vertices. Vertex number is used as
    // index in this array. The value '-1' of
    // colorArr[i] is used to indicate that no color
    // is assigned to vertex 'i'. The value 1 is
    // used to indicate first color is assigned and
    // value 0 indicates second color is assigned.
    var colorArr = Array(V).fill(-1);

    // Assign first color to source
    colorArr[src] = 1;

    // Create a queue (FIFO) of vertex numbers and
    // enqueue source vertex for BFS traversal
    var q = [];
    q.push(src);

    // Run while there are vertices in queue
    // (Similar to BFS)
    while (q.length != 0)
    {
        // Dequeue a vertex from queue
        var u = q[0];
        q.shift();

        // Return true if there is a self-loop
        if (G[u][u] == 1)
        return true;

        // Find all non-colored adjacent vertices
        for (var v = 0; v < V; ++v)
        {
            // An edge from u to v exists and
            // destination v is not colored
            if (G[u][v] == 1 && colorArr[v] == -1)
            {
                // Assign alternate color to this
                // adjacent v of u
                colorArr[v] = 1 - colorArr[u];
                q.push(v);
            }

            // An edge from u to v exists and
            // destination v is colored with same
            // color as u
            else if (G[u][v] == 1 && colorArr[v] ==
                                    colorArr[u])
                return true;
        }
    }

    // If we reach here, then all adjacent
    // vertices can be colored with alternate
    // color
    return false;
}

/* Driver code */
var G = [[0, 1, 0, 1],
            [1, 0, 1, 0],
            [0, 1, 0, 1],
            [1, 0, 1, 0]];

if (containsOdd(G, 0))
    document.write("Yes") ;
else
    document.write("No");


</script>

Output

No

Time complexity: O(V^2),The time complexity of the algorithm is O(V^2) as the outer loop runs V times and the inner loop runs V times for every iteration of the outer loop. 

Space complexity: O(V),The space complexity is O(V) as we use an array of size V to store the color of the vertices.

The above algorithm works only if the graph is strongly connected. We can extend it for the cases when graph is not strongly connected (Please refer this for details). In the above code, we always start with source 0 and assume that vertices are visited from it. One important observation is a graph with no edges is also Bipartite. Note that the Bipartite condition says all edges should be from one set to another.

If you like GeeksforGeeks and would like to contribute, you can also write an article using write.geeksforgeeks.org or mail your article to review-team@geeksforgeeks.org. See your article appearing on the GeeksforGeeks main page and help other Geeks.